Fraction to Decimal Converter
The Fraction to Decimal Converter is a user-friendly online tool, to convert Fraction to Decimal quickly.
Share This Converter
Related Converters
Fraction to Decimal Converter
A Fraction to Decimal Converter is a simple tool for converting fractions into decimal from. This is particularly valuable in cases involving accurate measurement, scientific calculations, or any activity that benefits most from decimal values for accuracy or ease of use.
About Fractions and Decimals
Fraction: A fraction is a way of showing a part of a whole number. It consists of two numbers: the top part (or numerator) of the fraction, and the bottom part (or denominator). That means the denominator is how many equal parts make up the whole and the numerator is how many parts we have. For example, in the fraction
As a decimal is 0.5.
How to convert Fraction to Decimal
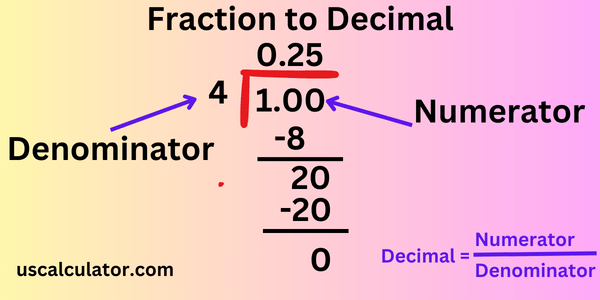
Here we have explained the simple way to convert fraction to a decimal, and also given an example that will make it easier for you to understand, you will use the steps given below.
We have given below the formula to convert any fraction into decimal.
Here we will divide to get the decimal equivalent.
Now write the fraction you want to convert. For example, let's use the fraction
.
Divide the numerator (top number) by the denominator (bottom number).
The result of this division is .
So, fraction is equivalent to the decimal .
Frequently Asked Questions (FAQ)
Q1. When I say terminating decimal, I mean it stops.
A decimal representation is a terminating one if it terminates after some number of digits. For example,
is a terminating decimal.
Q2. What is a repeating decimal?
A decimal is repeating if one or more digits repeat infinitely. For example,
The usual way that repeating decimals are written in mathematics involves a line over the repeating digit, like 0.3̅.
Q3. What clues do I have to judge whether a given fraction is going to be repeated or terminating decimal?
If after simplification it has a denominator with only 2 and/or 5 as its prime factors, then the fraction will have a terminating decimal. However, otherwise, it will be equivalent to a repeating decimal.
Q4. Is it possible to convert a mixed number into a decimal?
Yes, you can change mixed numbers into decimals even though fractional parts and whole numbers should be added first. For instance,
A Table for Fraction to Decimal Conversion
| Fraction | Decimal |
|---|---|
| 1/100 | 0.01 |
| 1/50 | 0.02 |
| 3/100 | 0.03 |
| 1/25 | 0.04 |
| 1/20 | 0.05 |
| 3/50 | 0.06 |
| 7/100 | 0.07 |
| 2/25 | 0.08 |
| 9/100 | 0.09 |
| 1/10 | 0.10 |
| 1/5 | 0.20 |
| 1/4 | 0.25 |
| 3/10 | 0.30 |
| 2/5 | 0.40 |
| 1/2 | 0.50 |
| 3/5 | 0.60 |
| 7/10 | 0.70 |
| 3/4 | 0.75 |
| 4/5 | 0.80 |
| 9/10 | 0.90 |
| 1/1 | 1 |