Arcsine calculator
Use this Arcsine calculator to easily and quickly calculate trigonometry functions in degrees or radians. Supports input of decimal numbers (1, 0.5, 0, -0.5 -1, etc.)
arcsin() = degrees
arcsin() = radians
Share This Calculator
Related Calculator
Table of Contents
An arcsine calculator: what is it?
A mathematical instrument called an arcsine calculator, often referred to as an inverse sine calculator, is used to determine the angle whose sine is a given number. Put more simply, it facilitates the reversal of the sine function.
How to Use the Arcsine Calculator
Using the Arcsine calculator is simple. First, we will enter the value of the sine in the calculator. To get the matching angle we will enter the exact sine value we want to know. Now we will choose the appropriate unit of measurement. Now we have to decide whether we want to show the result in degrees or radians. Now we will click the “Calculate” button to start the calculation. Now we will be shown by the calculator the angle that matches the given sine value.
The Arcsine Function
An arcsine function written as arcsine(x) or sin^(-1)(x) is like the inverse of the sine function. It takes the sine value (x) as a sign and displays the angle that gives rise to that sine value. It’s like searching for a secret code to find a secret message.
Find an angle with arcsine
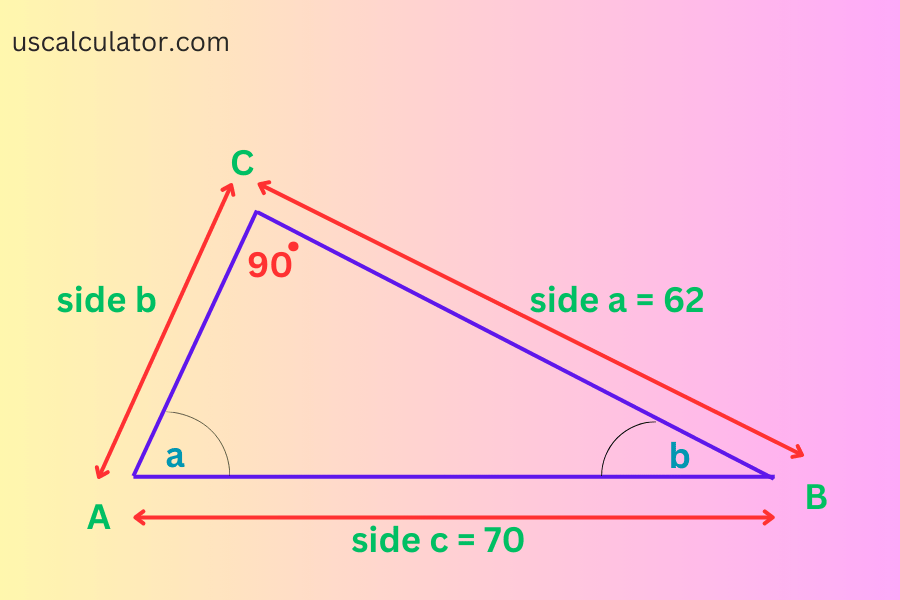
Now here we understand an example of the use of arcsine. To understand this, we have given below a picture of the isosceles triangle. In this figure, we have given two sides of known length.
The known side length is a = 62, the hypotenuse is c = 70. and a right triangle is shown at point C.
The arcsin of the inverse cosine function can be used to find the angle α at point A.
Now we first divide the opposite side and calculate the sine of α.
This gives sin (α) = a/c = 62/70 = 0.8857.
Now we will use the inverse function here. Using the inverse function, we get degrees and radians.
α = arcsine (0.8857) = 62.34° (1.088 radians).
Demystifying the Tool's Purpose
The arcsine calculator is essential in several domains, such as:
Finding angles in right triangles, solving triangles, and examining periodic functions are all part of trigonometry.
Physics: Understanding wave phenomena, analysing basic harmonic motion, and computing angles in projectile motion.
Engineering is the study of mechanical system analysis, force calculations, and structural design.
Mathematics: Determining inverse trigonometric functions, solving trigonometric equations, and investigating calculus ideas.
FAQ
1. What is the difference between arcsine and sine?
Sine is one of the percentiles of a right triangle that ties a triangle’s hypotenuse to the opposing side. A sine value is given, and a person may find its counterpart in terms of angle by the usage of the arcsine function.
2. Is there any sine value that could be calculated through the arcsine calculator?
Nevertheless, every permissible sine value – no matter whether it ranges from -1 to 1 – can be obtained using an arcsine calculator. It is however, very important to note that when using the calculator for any number outside this range it will generate an error.
3. How can I transmute degrees and radians?
Most calculators offer the capacity to convert between degrees and radians or one or the other is embedded in them. As an alternative, you might apply the subsequent formulas:
Radians to degrees: Coonan opines that to convert an angle in radians to that in degrees, it is necessary to multiply the value of the angle in radians by (180/π).
Converting degrees to radians: We know that, angle in degrees * (π/180) = angle in radians.
common arcsin values:
| X | arcsin(x)(degrees) | arcsin(x) (radians) |
|---|---|---|
| -1 | 90° | -π/2 |
| -√3 / 2 | -60° | -π/3 |
| -√2 / 2 | -45° | -π/4 |
| -1/2 | -30° | -π/6 |
| 0 | 0° | 0 |
| 1/2 | 30° | π/6 |
| √2 / 2 | 45° | π/4 |
| √3 / 2 | 60° | π/3 |
| 1 | 90° | π/2 |
| -1 | 90° | -π/2 |